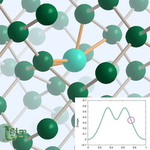
Snapshot of the movement of a Ge atom in a dumbbell interstitial position in silicon crystal. This movement includes an hexagonal interstital position as an on-the-way stable configuration. The evolution of the energy is plotted in the left-bottom box.
Research interests Diffusion of defects in Si and SiGe
Ab initio methods (DFT with
BigDFT and
ABINIT)
Monte Carlo algorithms (kinetic Monte Carlo on lattice)
Short CV
Since 2007 Research staff member at CEA/INAC Grenoble.
2006 post doctoral position in l'Université Catholique de Louvain (Belgium) in the team of
Xavier Gonze, working on
BigDFT
December 2005 PhD in physics, speciality in material sciences. Thesis directed by
Dr. F. Lançon [Grenoble - France].
October 2002 engineer degree from l'École Centrale (scientific and technical school) [Lyon - France].
Publications scientifiques
Selected papers:
-
Vacancy-mediated diffusion in biaxially strained Si
D. Caliste, K. Z. Rushchanskii, P. Pochet
Appl. Phys. Lett.,
Vol. 98, p. 031908 (2011). -
Daubechies wavelets as a basis set for density functional pseudopotential calculations
L. Genovese, A. Neelov, S. Goedecker, T. Deutsch, S.A. Ghasemi, A. Willand, D. Caliste, O. Zilberberg, M. Rayson, A. Bergman and R. Schneider
Journal of Chemical Physics,
Vol. 129, p. 014109 (2008). -
Sharing electronic structure and crystallographic data with ETSF_IO
D. Caliste, Y. Pouillon, M.J. Verstraete, V. Olevano and X. Gonze
Computer Physics Communications,
Vol. 179, p. 748-758 (2008) -
Germanium diffusion mechanisms in silicon: a first principle study
D. Caliste, P. Pochet, T. Deutsch and F. Lançon
Phys. Rev. B
Vol. 75, 125203 (2007). -
Vacancy-assisted diffusion in silicon: a three temperatures model
D. Caliste and P. Pochet
Phys. Rev. Lett.
Vol. 97, 135901 (2006). -
Thesis
manuscript:
Multi-scale simulations of defects diffusion in Si and SiGe semiconductors written in French.